

Next: Infinite body, cylindrical coordinate,
Up: Heat Equation. Transient Heat
Previous: Small-time GF, transient cases
The Heat Equation for transient 1-D Green's Functions in
cylindrical-radial coordinates is
Note that the cylindrical-radial Dirac delta function
 (r - r
(r - r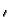 ) has vector arguments and has units of [
meters-2].
) has vector arguments and has units of [
meters-2].
Kevin D. Cole
2002-12-31